Derivation of various formulas for electromagnetic waves
*Since formula references jump around a lot, when you want to see the referenced formulas, we recommend that you view them in a duplicate separate window.
This course systematically derives various formulas for electromagnetic waves. It is recommended that you understand the entire flow.
・Fresnel's formula for p-polarized light (reflection and Snell's law are also derived along the way)
-
Parameter definitions and relationships
First, the definitions of the parameters of the electromagnetic wave will be shown.

Here, the permittivity and permeability in a vacuum are the following constants:

Based on the definition, the relationship between each parameter is expressed by the following equation.
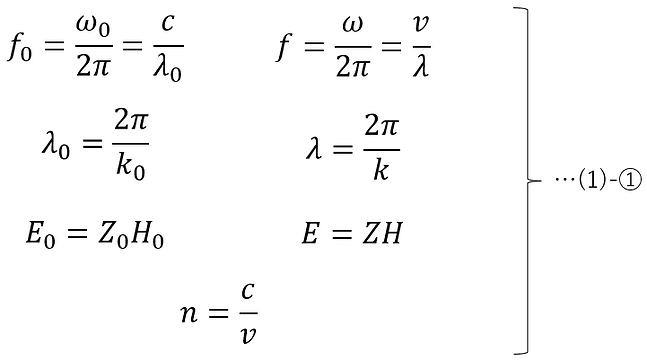
The meaning of each formula is shown below.
・The wavelength is the distance light travels in one vibration, while the wave number is the amount of vibration light makes per unit length, expressed in phase.
・The frequency is the number of times that light oscillates per second at a fixed position, and the angular frequency is the phase representation of this.
・Characteristic impedance is the ratio of the electric field to the magnetic field of the medium (transmission line or space) through which electromagnetic waves propagate.
・The refractive index is the ratio of the speed of light in a vacuum to the speed of light in a medium, and has a value specific to the medium. The refractive index is always greater than or equal to 1 (except for special cases such as metamaterials).
The relationship between the permittivity and permeability and each parameter is derived from Maxwell's equations [1], and the results are as follows:

In addition, the following relationship exists between the angular frequency in a vacuum and in a medium (this relationship is derived in (3)-⑥):
Applying (3)-⑥ to (1)-① and then integrating (1)-③ with this, we obtain the following equation:

In addition, for non-magnetic media, the magnetic permeability can be treated as follows:

In this case, (1)-④ can be further rewritten as follows:
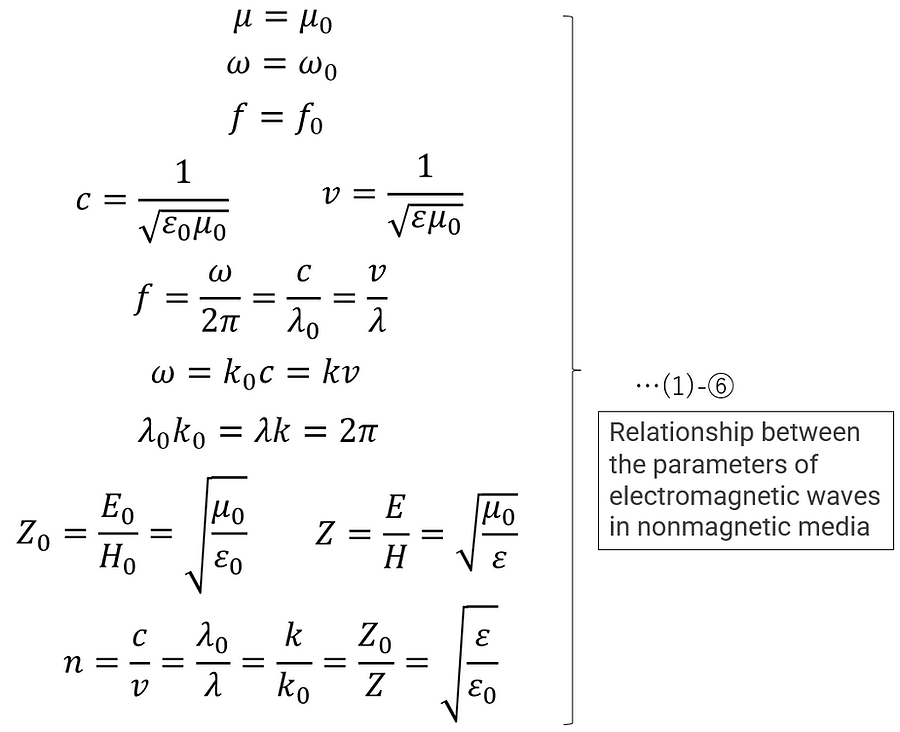
(1)-④ and (1)-⑥ are very important equations that show the relationship between the various parameters of electromagnetic waves in magnetic and non-magnetic media, respectively.
-
Mathematical expression of electromagnetic waves
Electromagnetic waves can be expressed as follows (only the results derived from Maxwell's equations are shown)[2]:
Electric field vector:
Magnetic field vector:

The relationship between the electric field and the magnetic field is as follows.
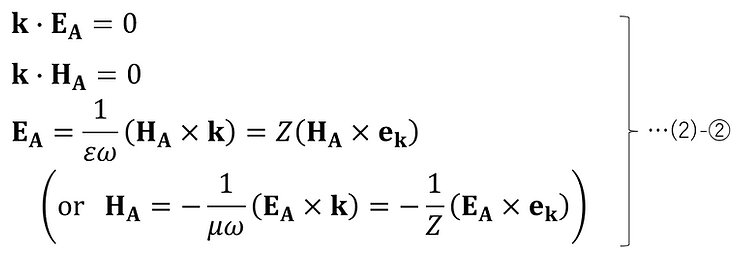
where each vector is a quantity defined as follows:
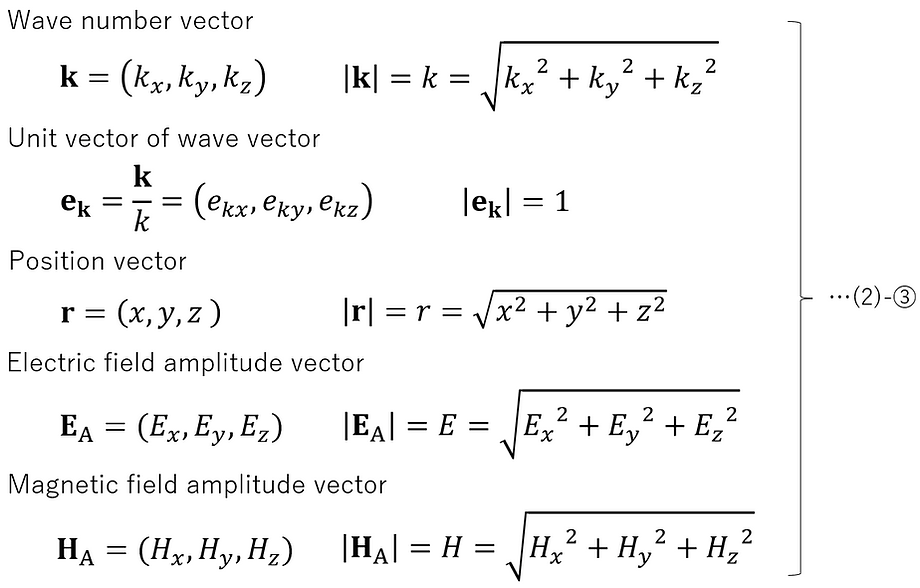
The relationship between the directions of the vectors shown in (2)-② can be illustrated as follows.
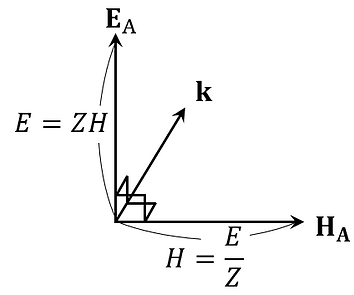
Here, the vector depth method can be expressed as follows:
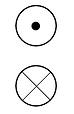
...From back to front
...From front to back
Using this, the relationship between the vector directions shown in (2)-② can be expressed as follows.
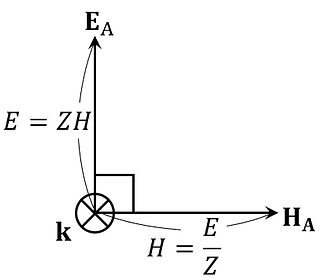
The electromagnetic wave represented by (2)-① can be illustrated as follows. Here, to avoid complicating the diagram, ekz =0, but the same consideration can be given to the case where ekz is not 0.

Moreover, the relationship between the phase lead and the electromagnetic wave lead can be illustrated as follows:
In this way, since the phases of the position advance and the time advance are in opposite directions, the signs of the position component and the time component are opposite.
-
Boundary conditions for propagation between media
As shown in the figure below, in the propagation of light between non-magnetic media, the z=0 plane is set as the boundary between the media, and we consider the transmitted and reflected light when an electromagnetic wave is incident on the boundary surface.

Here, the subscripts of each parameter have the following meanings:
i: Value for incident light
r: Value for reflected light
t: Value for transmitted light
The electric field and magnetic field of incident light, reflected light, and transmitted light are expressed as follows from (2)-①.
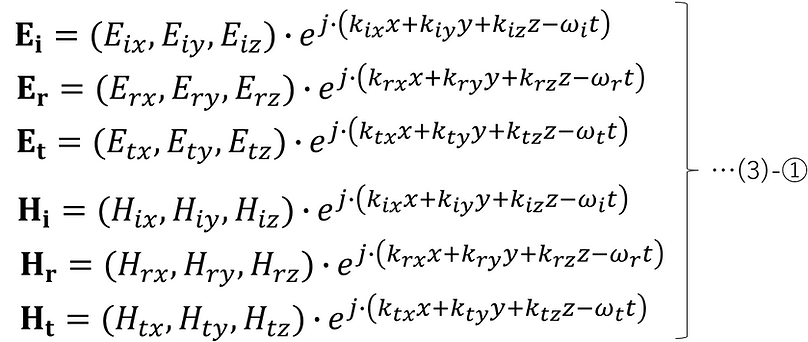
Now consider the boundary conditions of the electromagnetic field at the boundary surface.
Since the tangential component of the electric field is continuous at z=0,

Since the tangential component of the magnetic field is continuous at z=0,

For any x, y, and t, the conditions for (3)-② and (3)-③ to hold are

In this case, (3)-② and (3)-③ will be as follows.
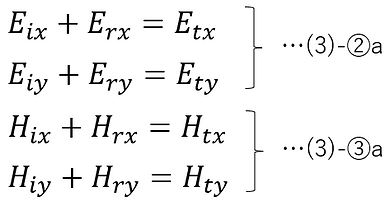
From (3)-④-3, the ω of incident light, reflected light, and emitted light will be expressed as a common ω without the subscript.
In addition, since ω does not depend on the medium, the following can also be said:
Here, there is no loss of generality in determining the y-axis in the direction where 𝑘𝑖𝑦 = 0.
In this case, from (3)-④-1, we get the following.
From this, if the y-axis is determined in the direction where 𝑘𝑖𝑦 = 0, it can be said that the incident light, reflected light, and transmitted light are all within the xz plane .
In addition, since the electric field and magnetic field can each be expressed as the sum of a component that vibrates parallel to the plane of incidence (p-polarized light) and a component that vibrates perpendicular to the plane of incidence (s-polarized light), we will consider these separately in the next chapter.
-
Fresnel's formula for p-polarized light
Consider the case where the electric field oscillates parallel to the plane of incidence (p-polarization) when light is incident on the boundary surface of a z=0 plane medium. In this case, the electric and magnetic fields of the incident light, reflected light, and transmitted light are expressed as shown in the figure below.

The subscripts 1 and 2 represent different media.
The directions of the electric and magnetic fields are illustrated according to (2)-②.
It is assumed that propagation occurs between non-magnetic, absorption-free media, and that (1)-⑥ is applicable.
Each vector shown in the figure can be expressed in component form as follows:
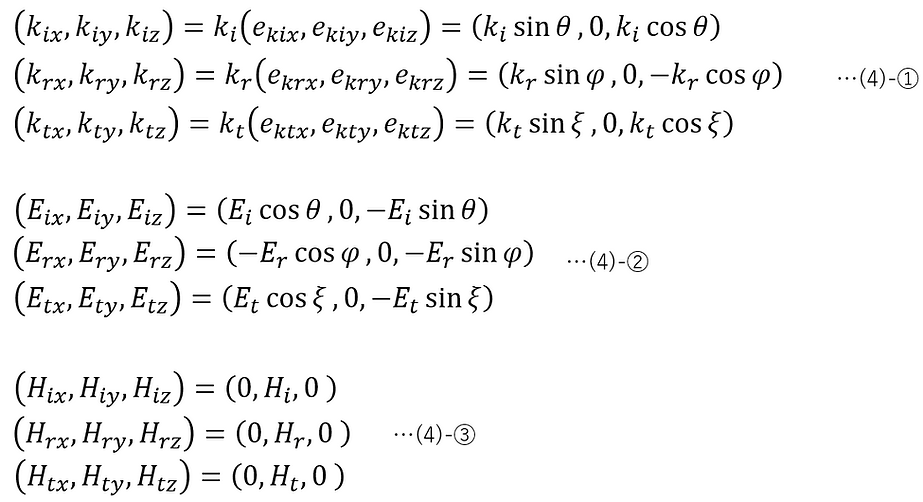
Here, from (1)-⑥,

from (4)-④-1,

In addition, from (3)-④-1 and (4)-①,

From (4)-⑤ and (4)-⑥, the following law of reflection and Snell's law can be derived.

Substituting (4)-②③ (electric field and magnetic field component expression for p-polarized light) into (3)-②a, ③a (boundary condition for electric field and magnetic field amplitude), and then applying (4)-④-2 (relationship between electric field and magnetic field amplitude) and (4)-⑦-1 (law of reflection), we obtain the following:
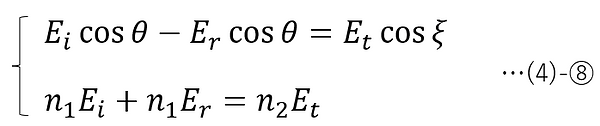
Therefore, if the amplitude transmittance is tp and the amplitude reflectance is rp , the following Fresnel formula (p-polarized light) can be obtained.

-
Fresnel's formula for s-polarized light
Consider the case where the electric field oscillates perpendicular to the plane of incidence (s-polarization) when light is incident on the boundary surface of a z=0 plane medium. In this case, the electric and magnetic fields of the incident light, reflected light, and transmitted light are expressed as shown in the figure below.

The subscripts 1 and 2 represent different media.
The directions of the electric and magnetic fields are illustrated according to (2)-②.
It is assumed that propagation occurs between non-magnetic, absorption-free media, and that (1)-⑥ is applicable.
Each vector shown in the figure can be expressed in component form as follows:
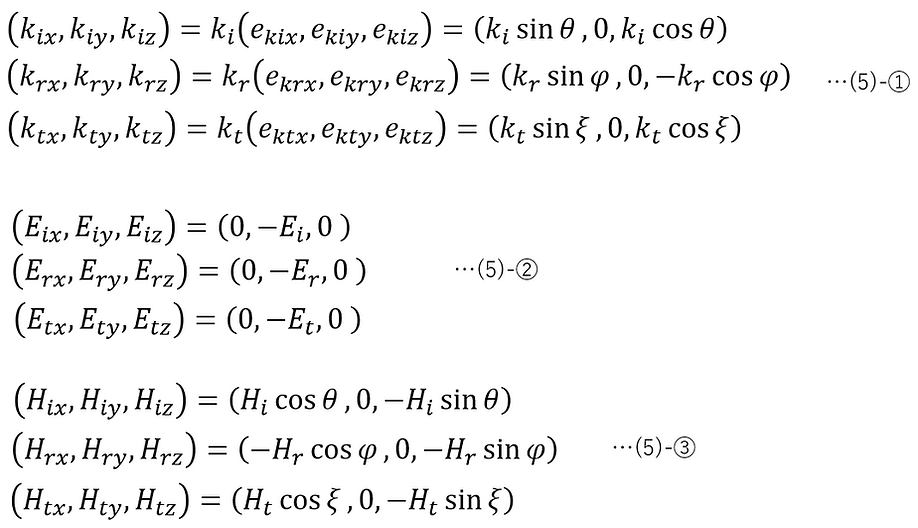
Here, from (1)-⑥,
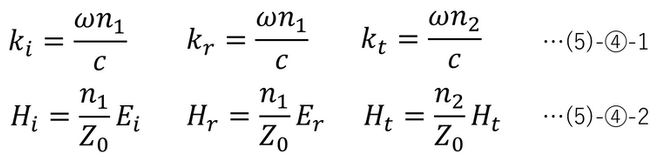
(5)-④-1,

In addition, from (3)-④-1 and (5)-①,

From (5)-⑤ and (5)-⑥, the following law of reflection and Snell's law can be derived.

Substituting (5)-②③ (electric field and magnetic field component expression for s-polarized light) into (3)-②a, ③a (boundary condition for electric field and magnetic field amplitude), and then applying (5)-④-2 (relationship between electric field and magnetic field amplitude) and (5)-⑦-1 (law of reflection), we obtain the following:
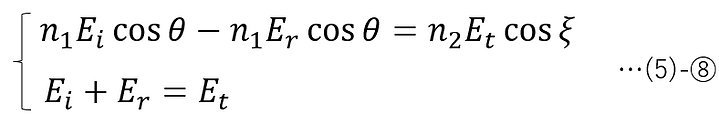
Therefore, if the amplitude transmittance is ts and the amplitude reflectance is rs, the following Fresnel formula (s-polarized light) can be obtained.

-
Transmittance and Reflectance
The volumes of the incident light, reflected light, and transmitted light passing through the infinitesimal region dxdy on the z=0 plane per unit time are dVi , dVr , and dVt , respectively. These are illustrated as follows.
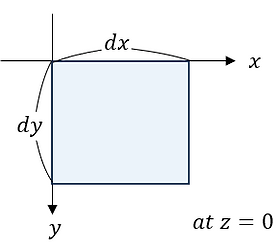
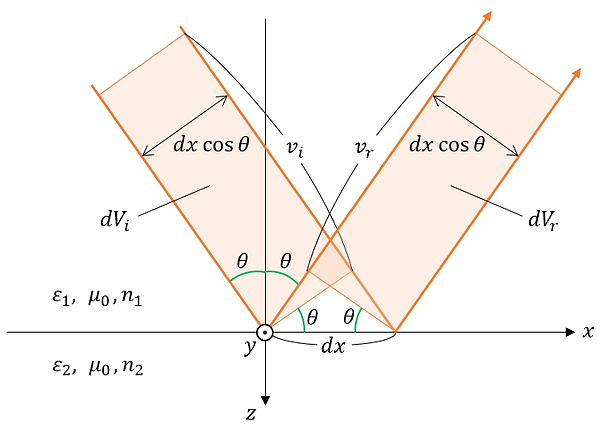
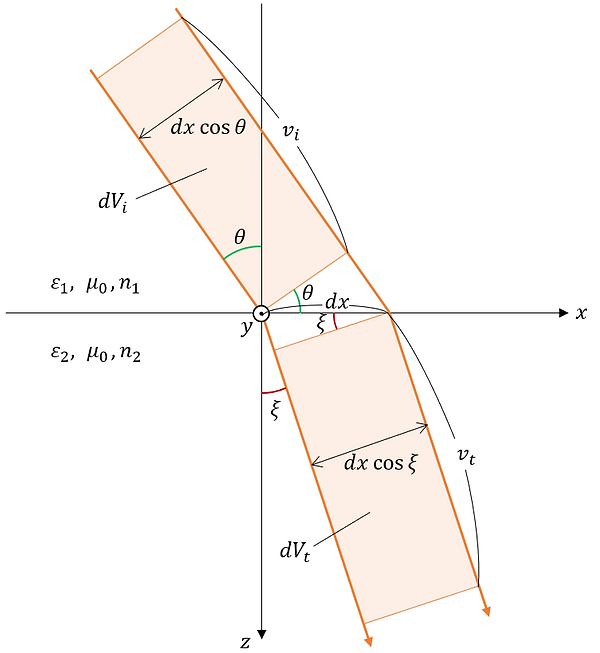
From the figure, dVi , dVr , and dVt can be expressed as follows.

The energy densities of the incident light, reflected light, and transmitted light are expressed as follows [3]:
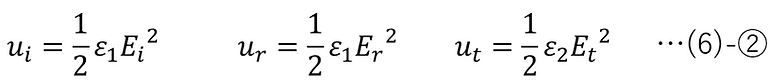
Therefore, from (6)-①, ② and (1)-⑤, the amount of energy of the incident light, reflected light, and transmitted light passing through the infinitesimal region dxdy on the z=0 plane per unit time can be expressed as follows:
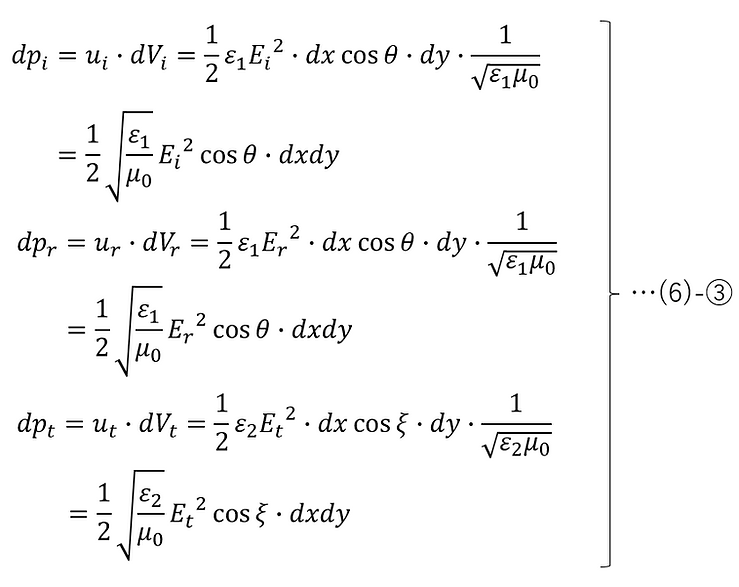
Therefore, the energy transmittance Tp and energy reflectance Rp of p-polarized light are as follows:

From (6)-④, the following calculation results confirm that the law of conservation of energy is satisfied.
At normal incidence:

Moreover, the energy transmittance Ts and energy reflectance Rs of s-polarized light are respectively as follows:

From (6)-⑦, the following calculation results confirm that the law of conservation of energy is satisfied.
For normal incidence, it is as follows. Since normal incidence of p-polarized light and normal incidence of s-polarized light are in the same state, it goes without saying that (6)-⑥ and (6)-⑨ have the same equation.

For the case of n1=1 and n2=2, if we graph (4)-⑨, (5)-⑨, (6)-④, and (6)-⑦, we get the results shown below.


When n1 > n2 , total reflection occurs as θ is increased, as will be explained later.
-
Brewster's Angle
As can be seen from the graph of the incidence angle dependence of energy reflectance shown in the previous chapter, p-polarized light is not reflected at a certain incidence angle. This angle is called the Brewster angle θB , and we will derive it.
In (4)-⑨, when rp =0,

(4)-⑦-2 (Snell's law)
(7)-① and ②,
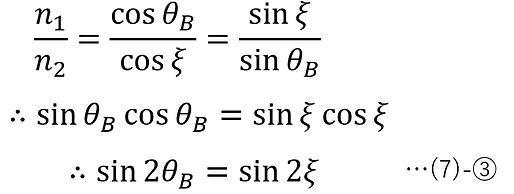
Here, the following angle conditions are considered:

The solution obtained at this time is as follows:
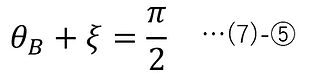
Again, from (4)-⑦-2 (Snell's Law),
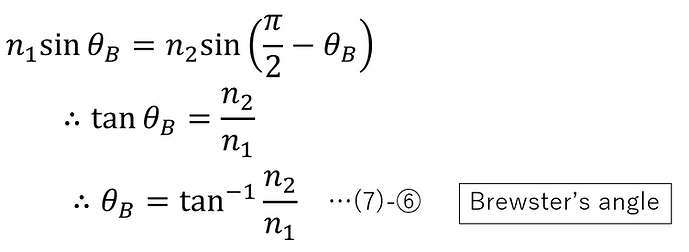
-
Total reflection and evanescent waves
When n1 > n2 , as the angle of incident light is increased, the exit angle ξ of the transmitted light becomes π/2 at a certain angle of incidence θ. The angle of incidence at this time is called the critical angle, and according to (4)-⑦-2 and (5)-⑦-2 (Snell's Law), it is as follows for both p-polarized light and s-polarized light.

At angles of incidence greater than this, Snell's law does not have a real solution ξ. In this case, all of the incident light is reflected. This is called total reflection. When n1 > n2 , the incidence angle dependence of the energy transmittance and reflectance is as follows:

Even under the condition of total reflection, if ξ is expanded to a complex number, Snell's law can have a solution. In other words, under the condition of total reflection, sinξ is a real value greater than or equal to 1, but ξ is a complex number.
The complex solution under the total reflection condition can be found as follows.

For p-polarized light, the electric field and magnetic field of the transmitted light under the total reflection condition are as follows, substituting (4)-①, ②, and ③ into (3)-① and applying (8)-②, (4)-④-2, (4)-⑤-2, (4)-⑥, and (4)-⑦-2.

For s-polarized light, the electric field and magnetic field of the transmitted light under the total reflection condition can be obtained by substituting (5)-①, ②, and ③ into (3)-① and applying (8)-②, (5)-④-2, (5)-⑤-2, (5)-⑥, and (5)-⑦-2.
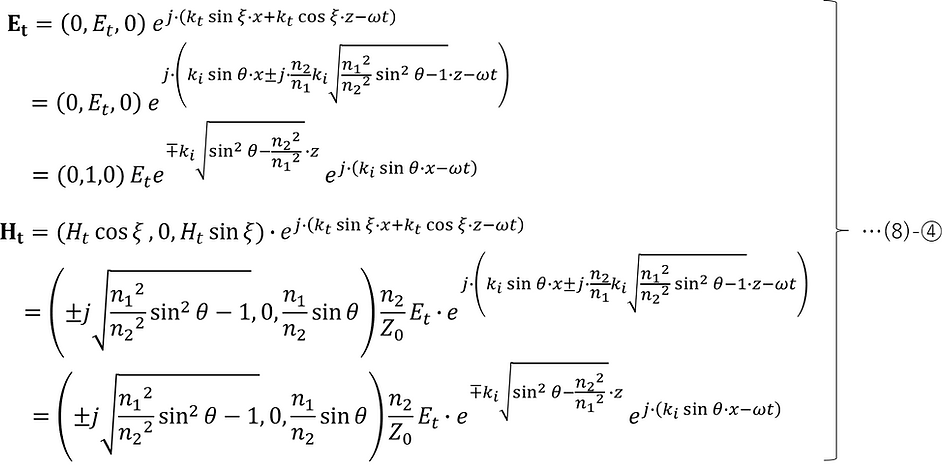
The result obtained is merely a mathematically obtained solution, but among the following z-dependent components that are common to both p-polarized and s-polarized light, the negative complex signs represent evanescent waves that actually exist physically (the positive complex signs do not exist physically).

This means that the amplitude decays exponentially with respect to z, and the distance from the interface (z=0) until the amplitude decays to 1/e (penetration depth) is expressed as follows (applying ③-⑧ by transforming the equation):

Regarding the effect of the penetration of evanescent waves on the amount of reflected light (energy reflectance), substituting (8)-② into (6)-④ and ⑦ gives Rp =1, Rs =1, which shows that there is no loss of light due to total reflection. However, when substituting (6)-④ and ⑦, the value of Tp does not become zero, and the denominator of Ts becomes zero, making it impossible to calculate. This is because evanescent light is not considered in the process of deriving the formulas for Tp and Ts .
-
Stokes' Relation
In (4)-⑨ and (5)-⑨, the amplitude reflectances of p-polarized and s-polarized light when entering medium 2 from medium 1 are rp and rs , respectively, and the amplitude transmittances of that are tp and ts , respectively . What is shown here applies to both p-polarized and s-polarized light, so the subscripts p and s are omitted, and the amplitude reflectance is expressed as r and the amplitude transmittance as t .
Also, the amplitude reflectance and amplitude transmittance when light is incident on medium 1 from medium 2 on the opposite side are denoted as r' and t', respectively.
Assume that neither medium 1 nor medium 2 has absorption.
In this case, the following electric field amplitude relationship in Figure 9-1 holds.

Next, if the reflected light in Figure 9-1 is directed in the opposite direction and made to enter the interface again, the electric field amplitude relationship in Figure 9-2 below holds true.
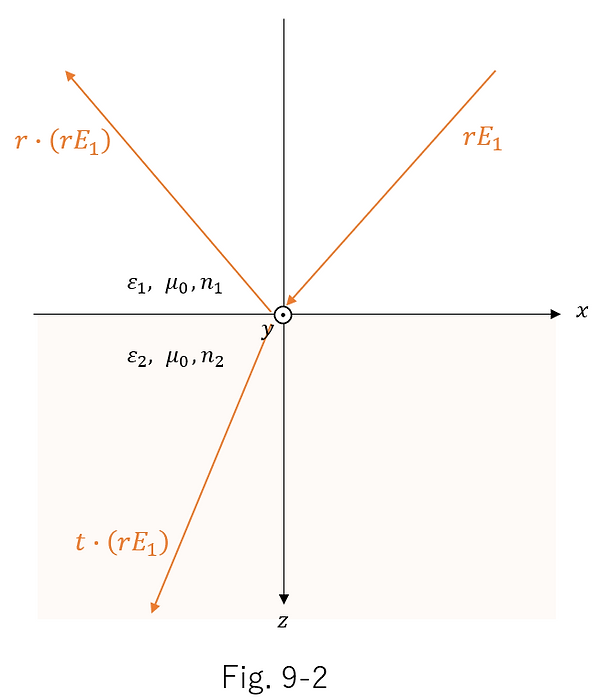
Furthermore, if the transmitted light in Figure 9-1 is directed in the opposite direction and incident on the interface again, the following electric field amplitude relationship in Figure 9-3 holds true.
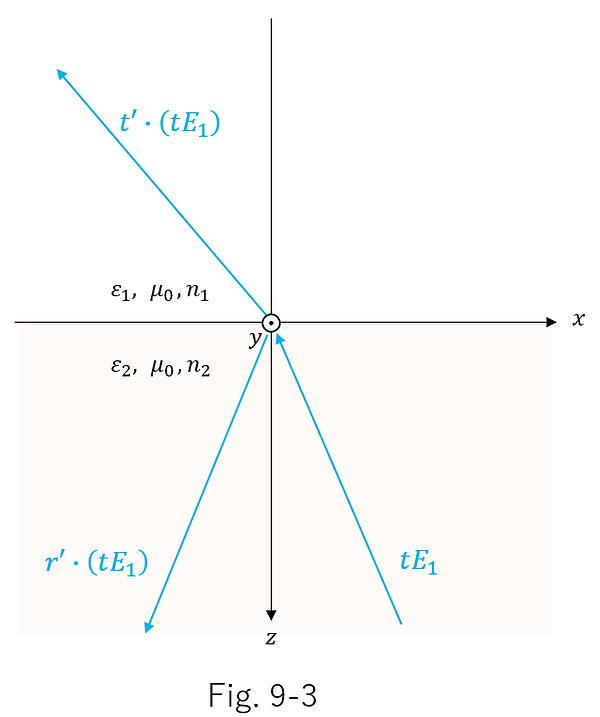
Also, if there is no absorption in the medium, the progression of light waves is reversible, so the following Figure 9-4 also holds true, in which the progression of all of the incident light, reflected light, and transmitted light in Figure 9-1 is reversed.
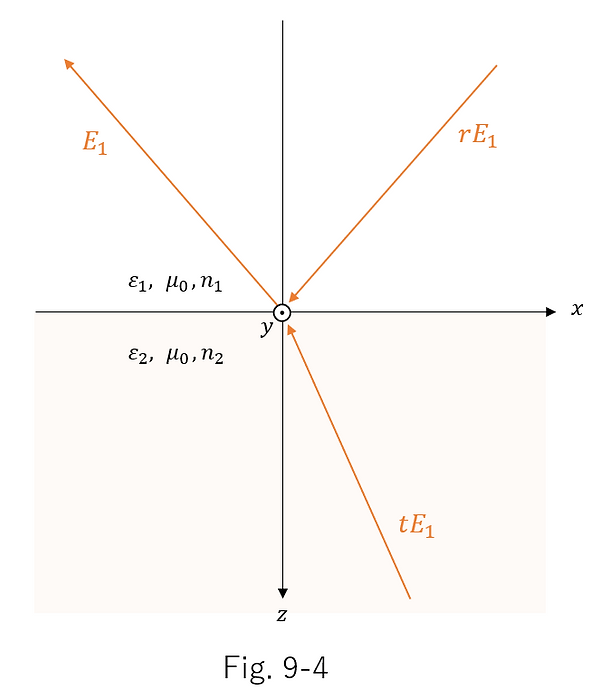
Here, Figure 9-5 below, which is a combination of Figures 9-2 and 9-3, has the same incident light as Figure 9-4, which means that the transmitted and reflected light should also be the same .

From this, the following equation holds:
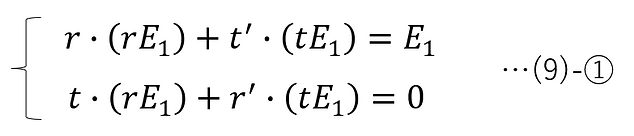
That is,
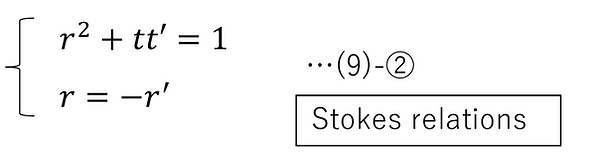
-
Extinction and Absorption Coefficients
Once again, (2)-① is shown below.
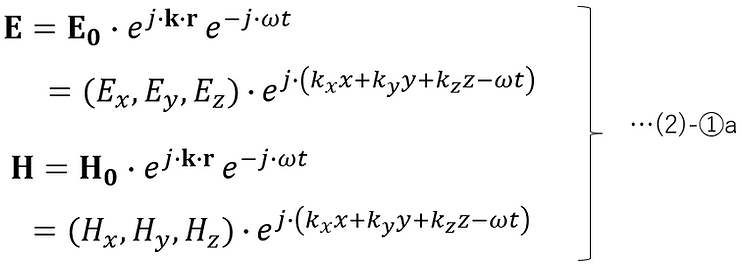
If the z-axis is taken in the same direction as the direction of light travel, then kx = ky = 0, and therefore k = kz , which can be expressed as follows.
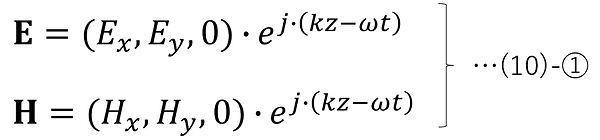
Here, from (1)-①,
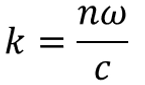
Now, replace the refractive index n by the complex refractive index shown below.
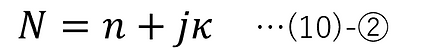
Here, κ is called the extinction coefficient.
At this time,

Therefore, if the electric field strength is I,

Here, the absorption coefficient α is defined as follows.

At this time,

This means that the electric field strength decays exponentially with z, where α is the depth from z=0 at which the strength falls off by a factor of 1/e.